‘The Stone Age didn’t end because of a shortage of stones.’
My Problem with 'avian' and 'swine' Flu
I really dislike the term "Swine Flu". Let's do a quick review on Influenza. There are many different types and strains of the virus, but the type that we are always referring to is Influenza A (They strains vary by the make up of certain proteins eg H1N1, H2N5, etc.) . Some of them are specific to different animals including birds, pigs, and humans. The virus can mutate one of two ways. It can have random error in its replication, or more than one strain of the virus can infect the same individual and they can mix together (reassortment). So let's say there is a pig specific flu going around in the pig community, but it isn't too bad--little piglet ts only need to stay home from school for a day before they get better. There is also a human specific flu going around in the human community that has a similar effect on little kids. These two flus aren't too bad because each population has their respective immunities. One day, a human with human flu sneezes on a pig and the pig is able to catch it. Then, her piglet comes home from school and gives her pig flu. The two flus undergo reassortment and make a new hybrid that can be spread to humans. Farmers Jane and John both die because they have no immunity. Now that you know how influenza works, please realize that the 1957 flu could have been called "duck flu" and the 1968 could have been remembered as "swine flu". My point is that one cannot label a flu by the animal it originated from because it is misleading. When you say "swine flu" one thinks you mean that a pig has the flu.
My Trip into the World of Calculus
For a long time, Calc has been a mystery to me. It has been referred to and talked about all around me; I feel like I have been missing out. For years, I have wondered what it is all about. The time to learn is now as I am taking Calc I at Eastern; although, I am beginning to wish I learned it on my own due to the disjointed and illogical nature of my lecturer. In an attempt to help study for what may very well be extremely easy quizzes (and to help me rationalize paying for and spending my entire mornings getting to and from this class) I am going to re-explain what we have done.
After 6 hours of class, this is what I have come up with:
First thing is first: Limits
Day 1: Say we had a function f(x)=x^2 (please note I am pretending by using the picture I found on google. Just pretend it is a parabola). At any point, we could say that as you approach any given value of x (lets say 2) you also approach the corresponding value of f(x) (in our case, 4!) That number is said to be the limit of a particular function as x approaches ___ (for us it was 2).
This is important because you need to use it when you want to find the equation for a tangent to a curve. Great. (The only practical example was when it is applied to mechanical physics in which they use the word 'velocity' to mean instantaneous velocity which bugs the senial-grandma-hell out of me.)
We are first going to find the slope then use the point-slope equation of a line to find the equation of the tangent. To find the slope of this tangent, we are going to find the slope of a secant whose pair of points includes the point from which we are drawing the tangent (P) . Slope is the change in values along the vertical axis divided by the change in values along the horizontal axis. rise/run (rise over run). Lets write that again replacing the words with variables 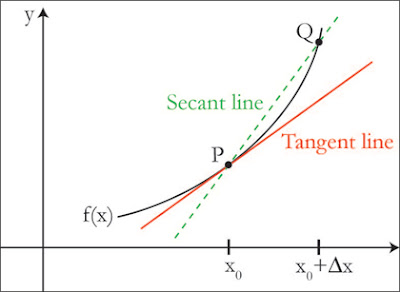 corresponding to the two items we have. What do we have?
corresponding to the two items we have. What do we have?
We have:
A curve
A tangent line
A secant line
The difference along the x axis is delta [x] and the difference along the y axis is [f(x+delta[x])-f(x)].
Therefore, rise/run is: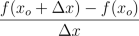
This equation gives the slope of any secant line; all one needs to do is fill in the variables with numbers.
Finally, we apply that thar limit thing to this. What we are trying to do is make the delta [x] as small as possible. In other words, we are going to make the point that is NOT the one we are finding a tangent on (Q) infinitesimally close to the one we are using (P). We do this by using a limit to say that as the difference between the x values of those two points gets closer to zero, our output of the equation will get closer to the slope of the tangent. Equationally, it looks like this: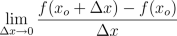
Problems will consist of a given function, lets say x^2, and the point to which we would like to make a tangent, (2,4) for us because it's easy. So let's plug stuff in: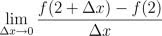
here, Xo was replaced with the x value of point P (2,4). Easy enough.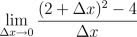
Now we know that f(x)=x^2 is the rule used to create the curve in the picture. In the equation, we choose another point by saying we are going to apply the function to the 'number' Xo plus some variable distance (written as Xo+Delta[x]). In this step, we plug that 'number' into the function to get (Xo+Delta[x])^2. We also replaced f(2) by what we know is the answer...4!
Now we simplify! We are going to end up with a squared Delta[x] plus four delta[x]s, no 4's all over a delta[x]. Extract a delta[x] from the numerator and cancel to get this: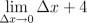
Now that everything is super simple, we can directly substitute the number that delta[x] is approaching (ZERO!) for delta x and what are we left with? The slope of the tangent line, which is 4!
Plug that slope into the point-slope form of a line along with point P to get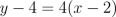
simplify to get the equation of the tangent line: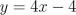
That was day 1. Yay.
Day 2: A few additions on yesterday's material about limits.
*A function may jump around (graphically) allowing it to have several limits that may seem counterintuitive at first. A function can have a limit on a part of the curve that approaches a number that it does not reach. For example, a curve may approach 3 as the x value approaches 1, but not include it. In fact, the value that is being approached does not have anything to do with the actual value at that point (in our example function, 1 may correspond to 4,321.7).
*Limits can be viewed as approaching from the right or from the left. If the limit of a function approaching from the left is different than the limit approaching from the right, the limit (not from a particular direction) is said not to exist.
*These are things you can do to limits: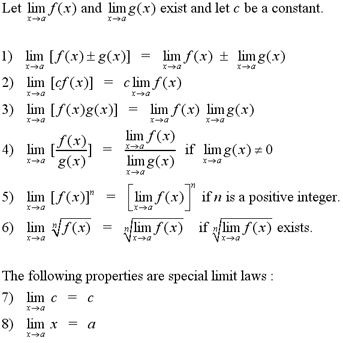
*It is possible for limits to approach infinity in either direction
Thats it for day 2...I had a half page of notes >.<
Day 3: Repeat Day One.
Ok, I bet you are all wondering how we did everything on day one! Well, we didn't. We used a different equation to do it...or something rather. Also, I did not feel like moving everything after I typed it, so it is going to stay where it is.
That's all for today. After all of that, I am going to be mad if I don't do well on this quiz. Also, please leave a comment and correct me if I screwed anything up...thanks.



